In this paper, we investigate the stability of following max-type difference equation 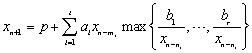 , where
, where ![]() , with
, with ![]() ,
, ![]() ,
, ![]() and
and ![]() , the initial values are positive. By constructing a system of equations and binary function, we show the equation has a unique positive equilibrium solution, and the positive equilibrium solution is globally asymptotically stable. The conclusion of this paper extends and supplements the existing results.
, the initial values are positive. By constructing a system of equations and binary function, we show the equation has a unique positive equilibrium solution, and the positive equilibrium solution is globally asymptotically stable. The conclusion of this paper extends and supplements the existing results.
| Published in | Applied and Computational Mathematics (Volume 5, Issue 2) |
| DOI | 10.11648/j.acm.20160502.13 |
| Page(s) | 51-55 |
| Creative Commons |
This is an Open Access article, distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution and reproduction in any medium or format, provided the original work is properly cited. |
| Copyright |
Copyright © The Author(s), 2016. Published by Science Publishing Group |
Difference Equations, Positive Solution, Convergence, Globally Stable
| [1] | El-Metwally H, “Global behavior of an economic model,” Chaos Solitons & Fractals, 33(3), 2007, pp. 994-1005. |
| [2] | El-Metwally H, El-Afifi M M, “On the behavior of some extension forms of some population Models,” Chaos Solitons & Fractals, 36(1), 2008, pp. 104-114. |
| [3] | Zhou L, Honghua H U, Liang C, et al, “Research on difference equation model in traffic flow calculation,” Journal of Chang chun University of Science & Technology, 2014, pp. 117-123. |
| [4] | Huang C M, Wang W P, “Applications of difference equation in population forecasting model,” Advanced Materials Research, 2014, pp. 1079-1080. |
| [5] |
Berenhaut K, Foley J, S. Stević S, “The global attractivity of the rational difference equation |
| [6] |
Berenhaut K S, Stević S, “The behavior of the positive solutions of the difference equation |
| [7] | Berg L, Stević S, “Periodicity of some classes of holomorphic difference equations,” Journal of Difference Equations and Applications, 12(8), 2006, pp. 827-835. |
| [8] | Iričanin B, Stević S, “Some systems of nonlinear difference equations of higher order with periodic solutions,” Dynamics of Continuous Discrete and Impulsive Systems Series, 13A (3-4), 2006, pp. 499–507. |
| [9] | Iričanin B, Stević S, “Eventually constant solutions of a rationa ldifference equation,” Applied Mathematics and Computation, 215, 2009, pp. 854-856. |
| [10] | Elabbasy E M, El-Metwally H A, Elsayed E M, “Global behavior of the solutions of some difference equations,” Advances in Difference Equations, 28(2), 2011, pp. 683-689. |
| [11] |
Elsayed E M, Iričanin B, Stević S, “On the max-type equation |
| [12] | Stević S, “Global stability of a max-type difference equation,” Applied Mathematics & Computation, 216(1), 2010, pp. 354–356. |
| [13] |
Sun T X, Xi H J, Han C H, “Dynamics of the max-type difference equation |
| [14] | Stević S, “On a symmetric system of max-type difference Equations,” Applied Mathematics and Computation, 219(15), 2013, pp. 8407-8412. |
| [15] | Stević S, “On some periodic systems of max-type difference equations,” Applied Mathematics and Computation, 218, 2012, pp. 11483–11487. |
| [16] |
Amleh A M, Georgiou D A, Grove E A, Ladas G, “On the recursive sequence |
| [17] | Fan Y, Wang L, Li W, “Global behavior of a higher order nonlinear diference equation,” Journal of Mathematital Analysis and applications, 299(1), 2004, pp. 113-126. |
| [18] |
Sun T X, He Q L, Wua X, Xi H J, “Global behavior of the max-type difference equation |
| [19] | Liu W P, Stević S, “Global attractivity of a family of non-autonomous max-type difference equations,” Applied Mathematics and Computation, 218(11), 2012, pp. 6297-9303. |
| [20] | Grove E A, Ladas G, Periodicities in Nonlinear Difference Equations, Vol. 4, New York: Chapman& Hall/CRC Press, 2005, pp. 2. |
APA Style
Han Cai-hong, Li Lue, Tan Xue. (2016). The Stability of High Order Max-Type Difference Equation. Applied and Computational Mathematics, 5(2), 51-55. https://doi.org/10.11648/j.acm.20160502.13
ACS Style
Han Cai-hong; Li Lue; Tan Xue. The Stability of High Order Max-Type Difference Equation. Appl. Comput. Math. 2016, 5(2), 51-55. doi: 10.11648/j.acm.20160502.13
AMA Style
Han Cai-hong, Li Lue, Tan Xue. The Stability of High Order Max-Type Difference Equation. Appl Comput Math. 2016;5(2):51-55. doi: 10.11648/j.acm.20160502.13
@article{10.11648/j.acm.20160502.13,
author = {Han Cai-hong and Li Lue and Tan Xue},
title = {The Stability of High Order Max-Type Difference Equation},
journal = {Applied and Computational Mathematics},
volume = {5},
number = {2},
pages = {51-55},
doi = {10.11648/j.acm.20160502.13},
url = {https://doi.org/10.11648/j.acm.20160502.13},
eprint = {https://article.sciencepublishinggroup.com/pdf/10.11648.j.acm.20160502.13},
abstract = {In this paper, we investigate the stability of following max-type difference equation , where , with , , and , the initial values are positive. By constructing a system of equations and binary function, we show the equation has a unique positive equilibrium solution, and the positive equilibrium solution is globally asymptotically stable. The conclusion of this paper extends and supplements the existing results.},
year = {2016}
}
TY - JOUR T1 - The Stability of High Order Max-Type Difference Equation AU - Han Cai-hong AU - Li Lue AU - Tan Xue Y1 - 2016/04/07 PY - 2016 N1 - https://doi.org/10.11648/j.acm.20160502.13 DO - 10.11648/j.acm.20160502.13 T2 - Applied and Computational Mathematics JF - Applied and Computational Mathematics JO - Applied and Computational Mathematics SP - 51 EP - 55 PB - Science Publishing Group SN - 2328-5613 UR - https://doi.org/10.11648/j.acm.20160502.13 AB - In this paper, we investigate the stability of following max-type difference equation , where , with , , and , the initial values are positive. By constructing a system of equations and binary function, we show the equation has a unique positive equilibrium solution, and the positive equilibrium solution is globally asymptotically stable. The conclusion of this paper extends and supplements the existing results. VL - 5 IS - 2 ER -